TCS NQT Free Mock Test
This test is designed to simulate the format and difficulty of the TCS National Qualifier Test (NQT), helping you prepare effectively. We have not added timer on student’s request to practice it little freely. Solutions to tough problems have been provided at the bottom.
Section 1: TCS NQT Numerical Ability Test (20 Qs, 25 mins)
Section 2: TCS NQT Reasoning Ability Test (20 Qs, 25 mins)
Section 3: TCS NQT Verbal Ability Test (25 Qs, 25 mins)
Section 4: TCS NQT Advanced Quant + Reasoning (15 Qs, 25 mins)
Mock Test is Done. But Are You Prepared?
Practice Smart and Track Progress with LearnTheta’s AI Practice Platform

TCS NQT Numerical ability Solutions
Sol : 1
To solve, first find the LCM of the divisors 15, 25 and 35
LCM(15,25,35) = 525.
Since the remainder is 7, the required number is: 525+7=532
Sol : 2
Calculate the number of students for each percentage:
Soccer only: 40% – 15% = 25% of 600
600×0.25=150
Basketball only: 30% – 15% = 15% of 600
600×0.15=90
Both sports: 15% of 600
600×0.15=90
Sol : 3
Calculate the discounted prices:
Jacket: 8000*(1-0.25)=6000
Shoes: 6000*(1-0.25)=4500
Add the discounted prices together:
6000+4500=10500
Add the 5% sales tax:
10500*(1+0.05)=10500*1.05 = 11025
Sol : 4
The formula for calculating the amount in compound interest is: $A = P \left(1 + \frac{r}{n}\right)^{nt}$
Plugging in the values: A = $ 1200 \left(1 + \frac{0.05}{1}\right)^{1 \times 2} =1323$
Sol : 5
Using the alligation method:
- Cost of Sugar A: 4
- Cost of Sugar B: 6
- Average cost of the mixture: 5
Alligation setup:
Difference from Average: Sugar A: 5−4=1 Sugar B: 6−5=1
The ratio of the quantities of Sugar A to Sugar B: Ratio of A to B=1:1
Since the total weight is 100 kg, the merchant should use 50 kg of each
Sol : 6
Let’s divide total required sugar by one cup capacity = (3/4) / (1/8) = 6
Sol : 7
Calculate the total score of the original 20 students = 20×75 =1500
Calculate the total score of the 5 new students = 5×85 = 425
Calculate the new total score = 1500+425=1925
New average = 1925/ 25 = 77
Sol : 8
Let the distance of each half be d.
- Time taken for the first half = d/6
- Time taken for the second half = d/4
- Total time for the race = t1+t2=d/6+d/4 = 5d/12
- Total distance for the race: Total distance=d+d=2d
- Overall average speed: Average Speed = Total Distance/Total Time = 2d/(5d/12) = 4.8 km/h
Sol : 9
- Calculate the work done by A and B in one day:
- Work done by A in one day = 1/10
- Work done by B in one day = 1/15
- Total work done by A and B together in one day = 1/10+1/15 = 1/6
- Work done by A and B together in 4 days: Work done in 4 days=4×1/6= 2/3
- Remaining work: Remaining work=1−2/3 = 1/3
- Time taken by B to finish the remaining work = Remaining work/B’s work rate=(1/3)/(1/15) = 5
Sol : 10
Rate of interest is weighted average = 0.4* 15 + 0.3 * 8 + 0.3 * 10 = 11.4%
Sol : 11
- Marked Price of the laptop: Rs. 40,000
- Discount Riya received:
- 20% of Rs. 40,000
- Discount = 0.20 × 40,000 = 8,000
- Cost Price for Riya = 40,000 − 8,000 = 32,000
- Selling Price of Riya to Neha (Profit = 30%):
- Profit = 0.30 × 32,000 = 9,600
- Selling Price = 32,000 + 9,600 = 41,600
- Marked Price for Neha (15% higher than Cost Price):
- Marked Price for Neha = 41,600 + (0.15 × 41,600) = 41,600 + 6,240 = 47,840
- Aditi bought the laptop at a 10% discount on the marked price:
- Discount = 0.10 × 47,840 = 4,784
- Cost Price for Aditi = 47,840 − 4,784 = 43,056
- Marked Up Price for Aditi (40% higher than Cost Price):
- Marked Up Price = 43,056 + (0.40 × 43,056) = 43,056 + 17,222.4 = 60,278.4
- Selling Price of Riya to Aditi (Extra paid = Rs. 2,500):
- Selling Price for Riya = 32,000 + 2,500 = 34,500
- Discount Given by Aditi:
- Selling Price = Marked Up Price − Discount
- 34,500 = 60,278.4 − Discount
- Discount = 60,278.4 − 34,500 = 25,778.4
- Percent Discount by Aditi:
- Percent Discount = (25,778.4 / 60,278.4) × 100 ≈ 42.8%
Sol : 12
Since the rate of work is same, 10 workers will take = 12*6/10 = 7.2
So workers will be finishing the task on 8th day (since it is not complete by 7th day)
Sol : 13
39 is divisible by 3 and 13
Sol : 14
- Cost Price (CP) of each phone:
CP = 12,000 / 50 = 240 - Marked Price (MP) of each phone:
MP = 300 - Selling Price (SP) after 20% discount:
- Discount = 20% of MP = 0.20 × 300 = 60
- SP after discount = MP − Discount = 300 − 60 = 240
- Total Selling Price for 30 phones:
Total SP = 30 × 240 = 7,200 - Total Cost Price for 30 phones:
Total CP = 30 × 240 = 7,200 - Profit:
Profit = Total SP − Total CP = 7,200 − 7,200 = 0
Sol : 15
Father’s Age = 40 years
Son’s Age = 40*2/5 = 16 years
Let y be the number of years until the father is twice the son’s age.
- At that time, the father’s age will be 40 + y.
- The son’s age will be 16 + y.
Set up the equation:
40 + y = 2(16 + y)
Solving the equation:
40 + y = 32 + 2y
40 – 32 = 2y – y
8 = y
Son’s age at that time:
Son’s age when father is twice as old = 16 + 8 = 24 years.
Sol : 16
Divide the equation by 2: $x^2 – 4x + 3 = 0$
$x^2 – 4x +3 = (x-3)*(x-1) = 0$
x = 1, 3
Sol : 17
Sugar : flour = 3 : 5 = 450 / x
x = 750g
Sol : 18
Time taken by train = 240/60 = 4 hr
Train will reach city B after 4 hours which is 1 PM
Sol: 19
Area = 1/2 * B * H = 1/2 * 10 * 6 = 30
Sol : 20
- Calculate the effective speed of the boat downstream: Effective Speed = Speed of Boat + Speed of Current = 15 km/h + 5 km/h=20 km/h
- Calculate the time taken to travel downstream: Time=Distance/ Effective Speed=60/ 20 =3 hours
Solutions: Advanced Quant and Reasoning test
Sol: 1
Using the identity $p^2+q^2=(p+q)^2−2pq$
$p^2+q^2=62−2k=36−2k$
40 = 36 – 2k
k = -2
Sol: 2
Identify the Sum and Product of the Roots: By Vieta’s formulas:
The sum of the roots = 10
The product of the roots = 24
Using Trial Factorizations or Testing Possible Root Pairs: We can quickly confirm that roots are 1, 2, 3, 4.
Calculate the Product of the Smallest and Largest Roots: The smallest root is 1, and the largest root is 4, so product is 4.
Sol: 3
3x – 7 < 2x + 5
3x – 2x < 7 + 5
Sol: 4
Just check all the options
Sol: 5
Mean = 89
Median = 88
Mode = 85
Sol: 6
Calculate the average for each store:
Store A: 290
Store B: 212.5
Store C: 355
Store D: 207.5
Sol : 7
Initial height of the tank = 8 (80% filled)
Volume of cuboid block = 2 * 2 * 4 = 16
Let’s assume increase in height as ‘h’
$ \pi \times (5)^2 \times h = 16 $
$ h = 16/(\pi \times 25)$ = 0.2 m
Sol : 8
Since Eric is at one of the ends, he can be either at the leftmost or rightmost position.
Fiona must be between Alice and Charlie, meaning Alice must be to the left of Charlie, forming the group as Alice, Fiona, Charlie.
Given that Bob cannot be next to Dana, if we place Eric on the left end, the arrangement looks like this: Eric, [ ], [ ], Dana, [ ], [ ].
The only possible arrangement that satisfies all the conditions is Eric, Alice, Fiona, Charlie, Bob, Dana.
In this arrangement, the person immediately to the right of Dana is Charlie.
Sol : 9
- Fix Gina in one position, and Jack must sit directly opposite her.
- Next, place Isla next to Henry. The arrangement could be: Gina, [ ], [ ], [ ], [ ], Jack, [ ].
- Place Isla next to Henry, leaving two spots.
- Since Lily cannot sit next to Mike, we can place Lily in one of the remaining spots and Kevin to the right of Lily.
- The only arrangement that satisfies all these conditions is: Gina, Isla, Henry, Mike, Lily, Kevin, Jack.
- In this arrangement, the person sitting immediately to the left of Mike is Henry.
Sol : 10
The coding follows a pattern where each letter is shifted by a certain number of positions in the alphabet:
- B → E (B + 3)
- R → U (R + 3)
- I → K (I + 2)
- G → W (G + 6)
- H → L (H + 3)
- T → V (T + 2)
Applying this to “CANDLE”:
- C → F (C + 3)
- A → D (A + 3)
- N → O (N + 1)
- D → Q (D + 6)
- L → O (L + 3)
- E → H (E + 2)
Sol : 11
For “GREAT”:
- G = 7
- R = 18
- E = 5
- A = 1
- T = 20
Thus, “GREAT” is coded as “7-18-5-1-20”.
Now for “FIND”:
- F = 6
- I = 9
- N = 14
- D = 4
So, “FIND” is coded as “6-9-14-4.”
Sol : 12
- Conclusion 1: Some pets are cats. (This does not necessarily follow; while it is possible, the statement does not provide direct evidence for this.)
- Conclusion 2: No dog is a cat. (This does not follow because it is possible that some dogs could be cats since not all mammals are dogs.)
- Conclusion 3: Some mammals are cats. (This follows because all cats are mammals, hence it is true.)
- Conclusion 4: Some pets are mammals. (This follows because since some mammals are not dogs, those mammals can still be pets.)
Thus, the valid conclusions are 1, 3, and 4.
Sol : 13
- Conclusion 1: Some herbs are flowers. (This does not follow because no flowers can be trees, and since herbs can be plants, they might not be flowers at all.)
- Conclusion 2: No plants are flowers. (This follows because all flowers are excluded from being trees and the statement doesn’t mention that any flowers can be part of plants.)
- Conclusion 3: Some trees are not herbs. (This follows because some plants are not herbs, and since all trees are plants, it implies that there are some trees that cannot be herbs.)
- Conclusion 4: All flowers are plants. (This does not follow since no flowers are trees, and plants include both trees and non-flowers.)
Thus, valid conclusions are 2 and 3.
Sol : 14
Project B has the nearest deadline (1 month) and requires only 30% of the team’s time, allowing for timely completion.
Project A, while high impact, requires 60% of the team’s time and has a longer deadline, making it less urgent.
Project C is low impact and has the farthest deadline, so it should be deprioritized.
Prioritizing Project B ensures that at least one project is completed on time while leaving some resources available for either Project A or C after that.
Sol: 15
Candidate X has more experience and technical skills, which are critical for a software development role. Hiring a more experienced candidate can lead to better performance, mentorship for other team members, and a quicker onboarding process.
While Candidate Y is less expensive, they may require more time to ramp up, which could delay project timelines and potentially lead to lower quality work.
If budget constraints are an issue, considering negotiation for Candidate X’s salary or evaluating budget allowances may be more beneficial than compromising on quality. Thus, the most prudent choice is to hire Candidate X for their proven capabilities, despite the higher salary.
If you are someone starting your aptitude preparation from scratch, we highly recommend going through a preparation structure we have built for you: https://www.learntheta.com/placement-aptitude-preparation/
Read more about LearnTheta’s AI Practice Platform: https://www.learntheta.com/placement-aptitude/
Fastest Way to Crack Aptitude Tests – LearnTheta’s AI-Practice!
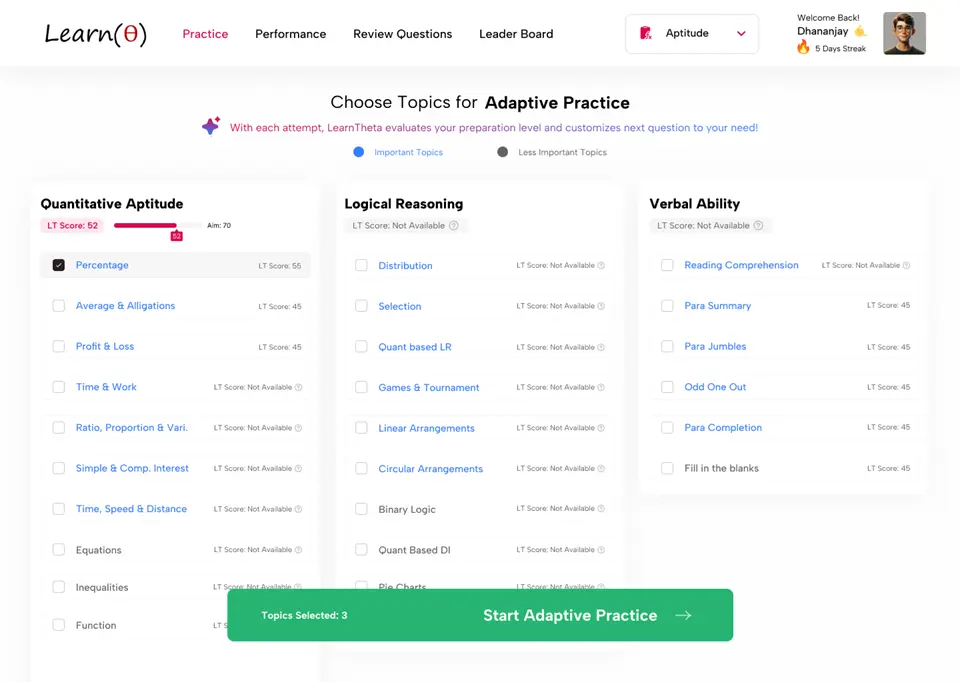
✅ All Topics at One Place

🤖 Adaptive Question Practice
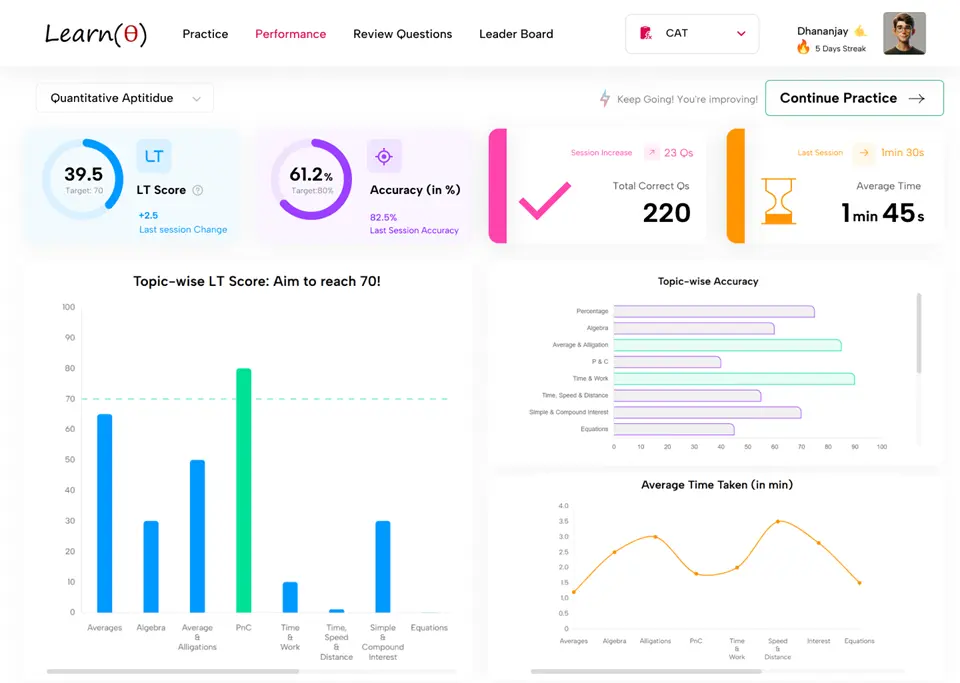
📊 Progress and Insights