Calendar and Clock: Reasoning Questions – Free Practice!
Topic-wise Aptitude Questions
Q. 1 “January 1, 2025” is a Wednesday. Fast forward an entire year, what day of the week will greet the new year on “January 1, 2026”?
Check Solution
Ans: A) Thursday
2025 is not a leap year, so it has 365 days. Hence, January 1, 2026, will be 365 days later, which is $365 \div 7 = 52$ weeks and 1 extra day.
So, the day will shift by 1 day: Wednesday + 1 = Thursday.
Q. 2 How many odd days are there in 1200 years?
Check Solution
Ans: B) 0
For 1200 years:
- 800 years (leap years) contribute (800÷4=200×2)=400(800 \div 4 = 200 \times 2) = 400(800÷4=200×2)=400 odd days.
- 400 years contribute 0 odd days (as the Gregorian cycle repeats).
Thus, $400 + 0 = 400 \div 7 = 0$ odd days.
Q. 3 Which of the following years is not a leap year?
Check Solution
Ans: C) 1900
A year is a leap year if:
- Divisible by 4.
- Not divisible by 100, unless divisible by 400.
1900 is divisible by 100 but not by 400, so it is not a leap year.
Q. 4 Picture a clock displaying the time 4:20. If you could freeze time and measure the angle formed between the hour hand and the minute hand, what would that angle be?
Check Solution
Ans: D) 10°
At 4:20:
- Hour hand’s position: $4 \times 30 + \frac{20}{60} \times 30 = 120 + 10 = 130°$.
- Minute hand’s position: $20 \times 6 = 120°$
Angle = 130°−120°=10°.
Q. 5 How many days are there between March 3, 2022, and March 3, 2023 (non-leap year)?
Check Solution
Ans: A) 365
2022 is not a leap year. From March 3, 2022, to March 3, 2023, there are 365 days.
Q. 6 Calendars repeat themselves every few years. Which of the following years will have the same calendar as 2024?
Check Solution
Ans: B) 2052
Leap years repeat every 28 years. Thus, 2024 + 28 = 2052.
Q. 7 How many times in a day do the hour and minute hands coincide?
Check Solution
Ans: C) 22
The hour and minute hands coincide once every hour, except at 12 o’clock. In a 12-hour cycle, this happens 11 times. Over 24 hours: $11 \times 2 = 22$
Q. 8 Think back to the dawn of the 21st century. When January 1, 2001, ushered in the new millennium, what day of the week did the world wake up to?
Check Solution
Ans: A) Monday
January 1, 2000, is Saturday (given). 2000 is a leap year.
So, January 1, 2001 = Saturday+2=Monday
Q. 9 If a clock shows 8:40, what will the time be in the mirror reflection?
Check Solution
Ans: A) 3:20
The time in the mirror = 12:00−8:40 = 3:20
Q. 10 If today is Sunday, what day will it be after 100 days?
Check Solution
Ans: D) Tuesday
100 days = $100 \div 7 = 14$ weeks + 2 days.
Sunday + 2 = Tuesday.
Practicing a Lot, Hmm…! But Are You Improving?
Adaptive Practice | Real Time Insights | Resume your Progress

Q. 11 If it was Sunday on 06 September 2009, then what was the day on 03 November 2011 ?
Check Solution
Ans: A)
Every non leap year will have 1 odd day and every leap year will have 2 odd days. Considering this find out total numbe of odd days for in between years and add that with days between initial and final year (excluding in between year since you have already calculated odd days for those years). You can also try other mental tricks like calculating difference between the days for the same year and then shifting it by odd days for every year
Q. 12 If it was Saturday on 14 August 2004, then what was the day on 26 August 2004 ?
Check Solution
Ans: D) Thursday)
Weekdays will repeat in a cycle of 7. So divide the differnece of days 26 – 14 = 12 by 7 to get remainder 5. Now count after Saturday for next 5 days
Q. 13 If day before yesterday was Thursday, then what day would it be after 11 days from today?
Check Solution
Ans: C) Wednesday)
Since a weekday repeats every 7 days, you need to divide no of days 11 +2 (to accommodate for day before yesterday by 7 to get remainder 6. Now count from Thursday for next 6 days
Q. 14 If it was Friday on 09 January 2009, then what was the day on 05 May 2009 ?
Check Solution
Ans: A)
Weekdays will repeat in a cycle of 7. Now calculate the no of days passed by counting days for in between months and in the intial and the final month. You will get total no of days as = 116. Divide it by 7 to get remainder 4. Now count from Friday for next 4 days
Q. 15 What day was it on 24 April 2024 ?
Check Solution
Ans: B)
Find out how many days passed till today from given date and divide the difference with 7 to get the remainder
Q. 16 When was Wednesday in the month of April 2024 ?
Check Solution
Ans: A)
Pick up one of the option and see what day falls on that date and then check for other options as the dates are next to each other
Refer Questions for next topic: https://www.learntheta.com/aptitude-questions-decision-making/
Refer Thousands of Reasoning Questions with Answers and Detailed Solutions
Fastest Way to Crack Aptitude Tests – LearnTheta’s AI-Practice!
✅ All Topics at One Place

🤖 Adaptive Question Practice
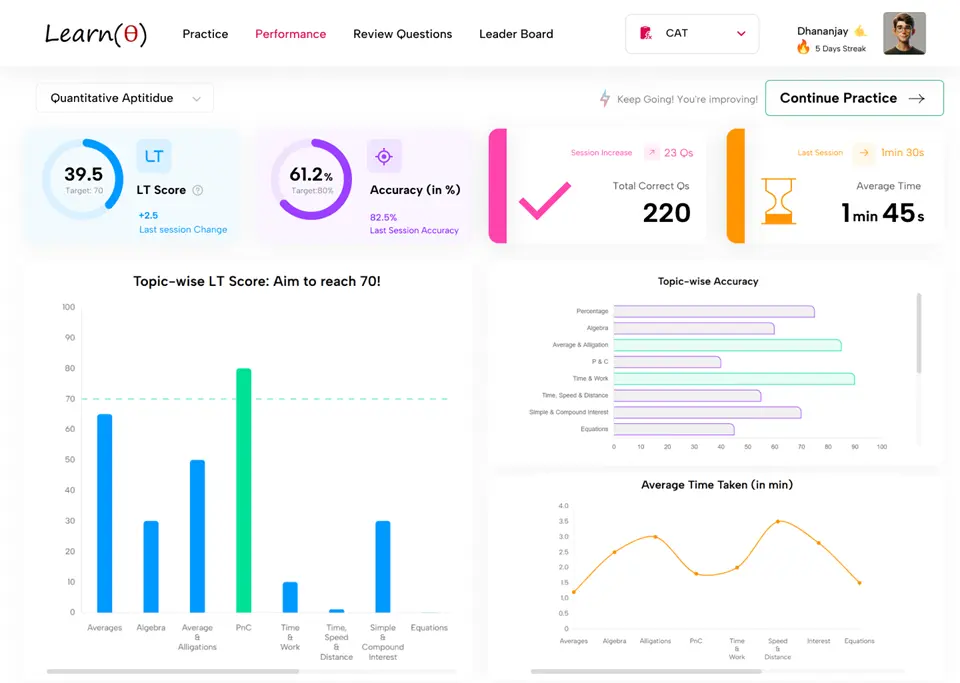
📊 Progress and Insights
bohot ache MCQ h