Mensuration -Formulas and Concepts for Aptitude

Basics

Mensuration is the branch of geometry that deals with the measurement of length, area and volume. Key terms used in mensuration are briefly explained below :-
- Length: The measurement of the longest side of a geometric figure.
- Area: The measure of the space enclosed by a geometric figure.
- Volume: The measure of the space occupied by a three-dimensional figure.
- Surface Area: The total area of the surface of a three-dimensional figure.
- Perimeter: The total distance around the boundary of a two-dimensional figure.

Triangles

The area of a triangle is represented by the symbol A. For any triangle, the lengths of the three sides are represented by a, b and c and the angles opposite these sides are represented by A, B and C respectively.
For any triangle in general –
- when the lengths of three sides a, b, c are given, \( \\ \) \(Area = \sqrt{s(s-a)(s-b)(s-c)} \\ \) where, \( s=\frac{a+b+c}{2}=\frac{p}{2} \) (This is called Heron’s formula.)
- when base (b) and altitude (height) to that base are given, \( \\ Area = \frac{1}{2} (base)(altitude)=\frac{1}{2}bh \)
For a right angled triangle –
- \( Area =\frac{1}{2}(Product \ of \ the \ sides \ containing \ the \ right \ angle) \\ \hspace{1cm} = \frac{1}{2}(base)(perpendicular) \)
For an equilateral triangle –
- \( Area = \frac{\sqrt{3}a^{2}}{4} \) where, “a” is the side of the triangle \( \\ \) The height of an equilateral triangle \( =\frac{\sqrt{3}a}{2} \)

Quadrilaterals

For any quadrilateral –
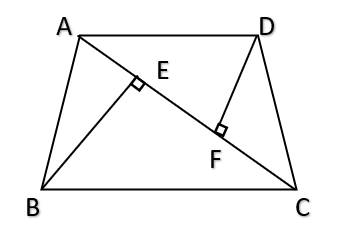
- \( Area \ of \ the \ quadrilateral = \frac{1}{2}(One \ diagonal) (Sum \ of \ the \ offsets \ drawn \ to \ that \ diagonal) \\ \) For the given figure, Area of quadrilateral (ABCD) \( = \frac{1}{2} (AC)(BE + DF) \\ \)
For a trapezium –
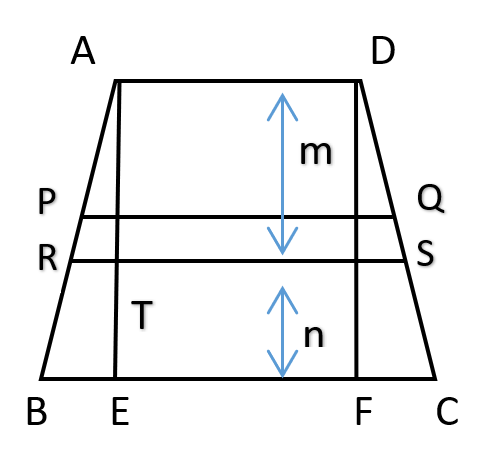
- \( Area \ of \ a \ trapezium = \frac{1}{2}(Sum \ of \ parallel \ sides) (Distance \ between \ them) \\ \) For the given figure, \( Area = \frac{1}{2}(AD+BC)(AF) \\ \)
For a parallelogram –
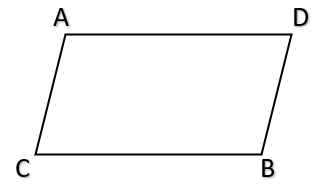
- \( Area = (Base) \times (Height) \) \( \\ \)
For a rhombus –
- \( Area =\frac{1}{2} (Product \ of \ the \ diagonals) \\ \hspace{1cm} = \frac{1}{2}(d_{1} \times d_{2}) \)
- \( Perimeter = 4(Side \ of \ the \ rhombus) \) \( \\ \)
For a rectangle –
- \( Area = (Length)(Breadth) = lb \)
- \( Perimeter = 2(l+b) \) , where l and b are the length and the breadth of the rectangle respectively \)
- \( Diagonal (d) = \sqrt{l^2+b^2} \) \( \\ \)
For a square –
- \( Area = (Side)^2 \)
- \( Area = \frac{1}{2} (Diagonal)^2 \)
- \( Perimeter = 4(Side) \)
- \( Diagonal = \sqrt{2} (side) \) \( \\ \)

Circle

\( Area \ of \ the \ circle = ar² \) where, r is the radius of the circle.
\( Circumference = 2 \pi г \)
\( Sector \ of \ a \ circle \ = Length \ of \ arc = \frac{\theta}{360°}(2πг) \\ Area = \frac{\theta}{360°}(πr^2) \) where \( \theta \) is the angle of the sector in degrees and r is the radius of the circle.
\(Area = (1/2)Ir \) where, l is length of arc and r is radius.
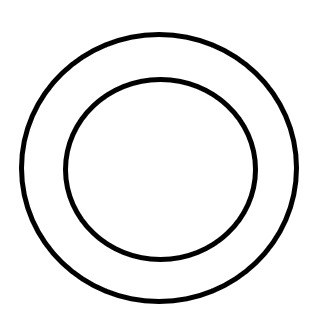
Ring – Ring is the space enclosed by two concentric circles. \( \\ Area = \pi R^2 – \pi r² = \pi (R+r)(R-r) \) where, R is the radius of the outer circle and r is the radius of the inner circle. \( \\ \)

Area and Volume of Solids

Solids are three-dimensional objects which, in addition to area, have volume also. For solids, two different types of areas are defined

Lateral surface area or curved surface area – lateral surface area is the area of the lateral surfaces of the solid.

Total surface area – Total surface area includes the areas of the top and the bottom surfaces also of the solid. Hence, Total surface area = Lateral surface area + Area of the top face + Area of the bottom face.

Cuboid

A right prism whose base is a rectangle is called a cuboid or rectangular solid.
If a and b are respectively the length and breadth of the base and h, the height of the prism, then –
- \( Volume = lbh \)
- \( Lateral \ Surface \ Area = 2(l+ b)h \)
- \( Total \ Surface \ Area = 2(l + b)h + 2lb\) \( \ = 2(lb + lh + bh) \)
- \( Longest \ diagonal \ of \ the \ cuboid = \sqrt{l² + b² +h²} \) \( \\ \)

Cube

A right prism whose base is a square and height is equal to the side of the base is called a cube.
If a is the edge of the cube, then –
- \( Volume = a³\)
- \( Lateral \ Surface \ Area = 4a^2 \)
- \( Total \ Surface \ Area = 6a^2 \)
- \( Diagonal \ of \ the \ cube = a \sqrt{3} \) \( \\ \)

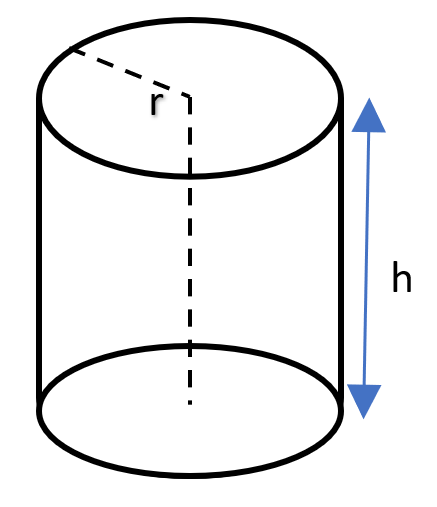
Cylinder

A cylinder is equivalent to a right prism whose base is a circle.
A cylinder has a single curved surface as its lateral faces.
If r is the radius of the base and h is the height of the cylinder, then –
- \( Volume = r²h \)
- \( Curved \ Surface \ Area = 2\pi rh \)
- \( Total \ Surface \ Area = 2 \pi rh+ 2 \pi r² \) \( \ = 2 \pi r(h + r) \) \( \\ \)
A hollow cylinder has a cross-section of a ring.
Volume of the material contained in a hollow cylindrical shell \( = \pi (R^2 – r^2)h \) where, R is the outer radius, r is the inner radius and h the height.

Pyramid

Cone

A cone is equivalent to a right pyramid whose base is a circle.
The lateral surface of a cone does not consist of triangles like in a right pyramid but is a single curved surface.
If r is the radius of the base of the cone, h is height of the cone and I is the slant height of the cone, then –
- \( l^{2} = r^{2}+h^{2} \)
- \( Volume = \frac{1}{3} \pi r^{2}h \)
- \( Curved \ Surface \ Area = \pi rl \)
- \( Total \ Surface \ Area = \pi rl+\pi r^2 \) \( \ = \pi r (l+r) \) \( \\ \)

Sphere

A sphere is a three-dimensional geometric object that is perfectly round in shape.
It is defined as the set of all points in space that are equidistant from a given point called the center.
If r is the radius of the sphere, then –
- \( Volume = \frac{4}{3} \pi г^3 \)
- \( Surface \ Area = 4 \pi r^2 \)
The of a hemisphere is equal to half the surface area of a sphere, i.e.,

Hemisphere

A hemisphere is a three-dimensional geometric shape that is half of a sphere.
It is formed by cutting a sphere along a plane passing through its center. This results in two equal parts, each resembling half of a sphere.
If r is the radius of a hemisphere, then –
- \( Volume = \frac{2}{3} \pi г^3 \)
- \( Curved \ Surface \ Area= 2\pi r^{2} \).

You can also refer following videos to grasp basics in more details

Practice

Refer Topic: Ratio and Proportion: https://www.learntheta.com/placement-aptitude-ratio-proportion/

Refer Aptitude Questions with Solutions on Mensuration: https://www.learntheta.com/aptitude-questions-mensuration/

Practice Aptitude Questions on Mensuration with LearnTheta’s AI Practice Platform: https://www.learntheta.com/placement-aptitude/